Sterren - College 8
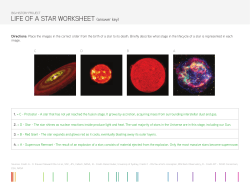
Sterren - College 8 Equation of state Ideal gas Degenerate gas LB 184 – 190 The evolution of stars LB 236 – 248 HR-diagram General considerations Low, intermediate and high mass stars + our sun Ideal gas ● Equation of state: ● Remember definition mean molecular weight: ● Hence: – with pressure due to ions ● Combined: ● Mass fraction of species i: ● Combined: and electrons: ● Ion pressure: ● Number density as function mass fraction: ● With Ai number of nucleons, approximate: ● Hence ● and: ● Electron pressure: ● For a completely ionised species/plasma, an atom of a given species j will yield zj electrons, hence ● As ● so: this gives: which is the number of free electrons per nucleon ● ● With X mass fraction of of Helium hydrogen, Y and Z of metals: Since for most elements, the number of protons in the nucleus is approximately equal to the number of neutrons: ● Using and ● we find: ● so if helium mass fraction go up, the pressure down or Degeneracy ● In classical physics, particles follow a continuous energy spectrum, while: ● ● ● ● ● ● Electrons have low momentum and obey Pauli's exclusion principle. At high density the velocity distribution does not follow a Maxwellian. For non-relativistic degenerate gas of electrons, pressure is *not* a function of temperature: This applies to white dwarfs and core of the sun after H-burning has stopped http://en.wikipedia.org/wiki/ Hertzsprung– Russell_diagram General considerations ● ● When a newly born star starts Hydrogen burning, it is at the Zero-Age Main Sequence (ZAMS) In the core, the completely ionised gas has a mean molecular weight: – ● ● ● ● where X and Y are the hydrogen and helium mass fractions During burning the mean molecular weight increases, and hence the pressure decreases The density increases/core contracts and (due to the virial theorem) the temperate rises So for two reasons the energy production then goes up: – higher temperatures give higher burning efficiencies, – nuclear burning takes place in a larger region. As a result, stars becomes a bit more luminous and their surface temperatures slightly hotter Mass is important < 0.5 Msun – after hydrogen burning a white dwarf is formed 0.5 Msun < M < ~ 10 Msun – first hydrogen and then helium burning, at the end a white dwarf is formed > ~10 Msun – burning up to the iron peak, end as supernovae+neutron stars or black holes Low-Mass Stars (M* ≤ 0.5M) ● ● ● ● lifetimes: 0.25Msun: 1012 yr, 0.08Msun: 1013 yr, longer than the age of the universe not massive enough to ever burn helium during a large fraction of their life they are fully convective. At the end of its life, a 0.08 M star has a hydrogen mass fraction of only 0.11. end as white dwarfs Evolution of our sun ● ● ● ● Again, energy production So for two reasons the energy production goes up, despite X going down: (i) higher temperatures give higher efficiencies, and (ii) nuclear burning takes place in a larger region. As a result, the sun becomes a bit more luminous and its surface temperature slightly hotter Due to the dependence of nuclear reaction rates on temperature, hydrogen is depleted faster in the centre of the star than in the outer core When hydrogen is depleted in the core, hydrogen burning takes place in a growing shell around the core.The sun continues to brighten, to finally become 2000 times more luminous as it is today. The outer regions of the star expand with a factor of ~100, become cooler and redder: the sun becomes a red giant for 109 yrs. ● ● ● ● ● ● ● When hydrogen in the core is exhausted the temperature is not high enough to start burning Helium (108 K). During contraction the core further heats up and the central(election) gas becomes degenerate – the gas pressure is independent of temperature. The temperature rises up to the point that Helium starts burning. When this happens the temperature shoots up (as the pressure stays constant the core does not expand), and during a short period (seconds) called the helium flash, helium in the core is burned. Virtually all of the energy released is used to undo the degeneracy and have the core expand. The luminosity of the star does not change appreciably. The star then stabilises and it is then located on the 'horizontal branch'. It consists of Helium burning core with a Hydrogen burning shell. After 108 yrs, the core stops burning Helium and largely consists of Carbon, with shells of He and H burning. The core contracts, heats up and the star climbs up the asymptotic supergiant branch and becomes a supergiant. Subsequently a white dwarf mainly composed of Carbon with mass 0.6 solar mass forms. The outer expanding shell is visible as a planetary nebula – T~10,000K, expansion speed 10 km/s, lifetime ~5 x 104 yrs. http://skyserver.sdss.org/dr1/en/astro/stars/stars.asp Evolution of massive stars (> 10 solar masses) After main sequence hydrogen burning a sequence of burning stages: ● At this late stage the star is a supergiant with up to a 10 6 solar luminosity and 1000 solar radii ● ● ● The iron core can not fuse further to produce more energy. Further contraction increases the temperature up to a point that photons can dissociate iron. An example: This takes away energy, accelerating the collapse of the core. Even helium can then dissociate: ● and the core will then mainly consist of protons, neutrons and free electrons ● Continued collapse enables 'neutronization': ● ● ● ● As the electrons disappear, the pressure drops and the core further collapses and a neutron star or black hole (core > 3 solar masses) is created. Note that outside the core not all iron has been destroyed As neutrons are fermions (half integer spin), the Pauli exclusion principle applies, limiting the density of the core. The collapsing material rebounces creating a supernova – which is as luminous as an entire galaxy During the supernova explosive nucleosynthesis takes place: the many neutrons can combine with heavy elements to form heavier elements than iron. Although rare (1 out of 1000 stars is heavier than 15 solar masses), the supernovae are very important as they enrich the ISM with heavy elements http://esoads.eso.org/abs/1967ARA%26A...5..571I
© Copyright 2025