Download Report

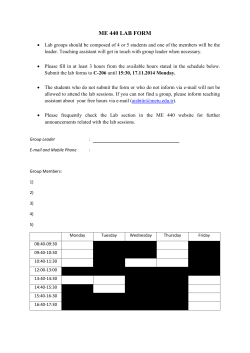
S-1a, Summer 2006
1
Harvard Summer School
Math S-1a ◊
Calculus I
◊
Summer 2006
Class Meetings
Monday through Friday 10 AM – 10:50 (Section 1) or 11 AM – 11:50 (Section 2).
Location: Science Center 507. Classes start Tuesday, June 27.
Afternoon Sessions
The daily afternoon sessions, conducted by Teaching Fellows (TFs), are an integral part of
the course. Generally, the theoretical foundations of the material will be developed in class. In
the sessions, the Teaching Fellows will conclude the coverage of the theory, show alternative
approaches to the material, and, most importantly, work examples.
One-hour sessions will be offered at 1 PM and 2 PM. Sessions will start Wednesday, June 28.
Instructor
Instructor:
Prof. Otto Bretscher
e-mail:
otto@post.harvard.edu
Office:
Science Center 435 (tentative)
Office Hours: Monday through Friday 9 - 10 AM, and by appointment.
Phone
(857) 928-8075
Web Site:
www.colby.edu/~obretsch
Exams
There will be two exams in class, on Wednesday, July 12, and on Wednesday, August 2. Time
and location of the Final Exam will be announced in class.
Problem Sets
Problem Sets will be assigned in class; on average, there will be three assignments a week. Your
homework will be collected and returned in the afternoon sessions.
Grades
Course grades will be based upon the two in-class exams (20% each), the final exam (40%),
and homework (20%). You can earn a few extra points for active class participation.
Text/Lecture Notes
The (optional) text for the course is Thomas’ Calculus by Weir et al., published by Pearson.
Detailed lecture notes will be distributed in class, and all the homework will be from handouts.
Calculators
A graphing calculator is not required for this course, and calculators will not be allowed in the
exams.
Prerequisites
I expect a fine working knowledge of algebra, trigonometry, and exponential functions;
there will be no time to review these topics in class. However, no previous exposure to calculus
is required. In my experience, your success in this course will depend on your mastery of the
prerequisites (algebra in particular) and on your willingness to work hard.
Placement
Eligibility for math courses through Math S-1ab is determined by the results of a placement test
that is given online as well as on-campus. The on-campus placement test is offered on Monday,
S-1a, Summer 2006
2
June 26 at 4 PM in SC E. A late placement test is offered on Tuesday, June 27 at 4 PM in SC E.
If you have questions regarding your placement, you can talk to Srdjan Divac on June 27 through
June 30, 1:30 – 3:30 PM in SC 435.
Calculus after Hours
There is a lab for this course from 7 PM to 9 PM on evenings before homework is due
and on evenings before scheduled exams. One of the instructors will be available to help
you with the homework and explain ideas that are troubling you. Many of our students in
the past have found this program to be extremely helpful, a “life saver”. Check it out!
Problem Sets
The due dates are tentative
Problem Set 1: Section 1.1, Problems 2, 10, 16, 20, 24, 28, 30, 32. Due Friday, June 30
Problem Set 2: Section 1.2, Problems 4, 12, 34, 36, 40, 42, 44, 50. Due Monday, July 3
Problem Set 3: Section 2.1, Problems 4, 10, 18, 22, 34, 38, 42, 52. Due Thursday, July 6
Problem Set 4: Section 2.2, Problems 2, 8, 16, 20, 26, 34, 40, 48. Due Friday, July 7
Problem Set 5: Section 3.1, Problems 4, 22, 36, 40, 42, 50, 54, 66. Due Tuesday, July 11
Problem Set 6: Section 3.2, Problems 2, 6, 8, 10, 12, 14, 16. Due Thursday, July 13
Problem Set 7: Section 3.3, Problems 8, 16, 20, 24, 38, 44, 52, 60. Due Friday, July 14
Problem Set 8: Section 4.1, Problems 4, 10, 14, 16, 18, 20, 26, 28. Due Tuesday, July 18
Problem Set 9: Section 4.2, Problems 10, 12, 16, 20, 22, 30, 34, 42. Due Wednesday, July 19
Problem Set 10: Section 4.3, Problems 22, 24, 30, 32, 34, 36, 40, 44. Due Thursday, July 20
Problem Set 11: Section 4.4, Problems 2, 6, 8, 10, 12, 16, 18, 22. Due Friday, July 21
Problem Set 12: Section 5.1, Problems 2, 10, 20, 34, 36, 40, 42, 44. Due Monday, July 24
Problem Set 13: Section 5.2, Problems 2, 12, 20, 22, 28, 30, 32, 34. Due Wednesday, July 26
Problem Set 14: Section 5.3, Problems 4, 10, 12, 16, 20, 26, 32, 34. Due Thursday, July 27
Problem Set 15: Section 5.4, Problems 4, 24, 32, 38, 42, 48, 52, 58. Due Friday, July 28
Problem Set 16: Section 6.1, Problems 8, 16, 24, 26, 30, 36, 38, 40. Due Tuesday, August 1
Problem Set 17: Section 6.2, Problems 4, 20, 28, 36, 44, 48, 52, 66. Due Friday, August 4
Problem Set 18: Section 6.3, Problems 4, 6, 10, 14, 16, 22. Due Tuesday, August 8
Problem Set 19: Section 8.1, Problems 2, 6, 12, 18, 20, 22. Due Thursday, August 10
Problem Set 20: Section 8.2, Problems 2, 4, 6, 8, 12, 16. Due Friday, August 11
S-1a, Summer 2006
Tentative Syllabus
Sections marked (*) are covered in Calculus II
Part I : Concepts of Calculus
Chapter 1: Introduction to the Derivative
1.1 What is Speed?
1.2 Rules of Differentiation
Chapter 2: Limits and Continuity
2.1 More on Limits
2.2 Continuity and Differentiability
2.3 A closer look: Epsilon and Delta (*)
Chapter 3: Using the Derivative to Analyze a Function
3.1 Maxima and Minima
3.2 The Mean Value Theorem
3.3 Concavity
Chapter 4: Introduction to the Integral
4.1
4.2
4.3
4.4
Riemann Sums
The Definite Integral
Antiderivatives and The Fundamental Theorem of Calculus
More on Antiderivatives
Part II : Techniques of Calculus
Chapter 5: Basic Rules of Calculus
5.1
5.2
5.3
5.4
Trigonometric Functions
Leibniz Notation, Chain Rule, and Implicit Differentiation
Integration by Substitution
Product Rule, Quotient Rule, and Integration by Parts
Chapter 6: Exponential and Logarithmic Functions
6.1
6.2
6.3
6.4
The Natural Logarithm
Exponential Functions and their Derivatives
Exponential Growth and Decay
More on Inverse Functions (*)
Chapter 7: More Techniques of Integration
7.1 Partial Fractions, Trigonometric Substitutions, etc. (*)
7.2 Numerical Integration (*)
Part III: Applications of the Calculus
Chapter 8: Applications of the Derivative
8.1
8.2
8.3
8.4
Constrained Optimization.
Related Rates
Newton’s Method (*)
L’Hôpital’s Rule (*)
(Chapter 9: Applications of the Integral; this material is covered in Calculus II)
3