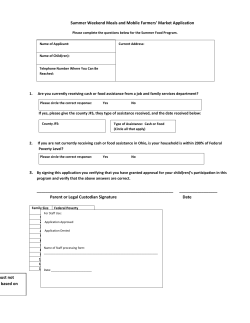
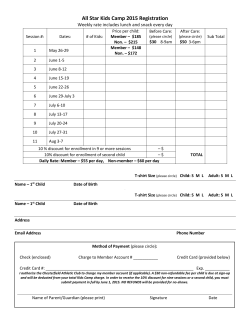
Understand the problem.
Grade 11 Mathematics Page 1 of 3 Applications of Sine and Cosine Law (hints only) Date: B 1. In parallelogram ABCD, the lengths of the sides are 22 cm and 65 cm. If the length of the diagonal is 77 cm, determine the area of the parallelogram. A common question is, “Which diagonal?” It’s important to realize that it doesn’t matter! 65 cm C 77 cm ? 22 cm or is BD 77 cm ? A Why? D 2. Three circles with centres A, B, and C are mutually tangent and no circle lies inside of another circle. The circle with centre A has radius 3 cm and the circle with centre B has radius 5 cm. If ∠BAC = 60° , determine the radius of the third circle and the area of ∆ABC . 3 A 5 B K 60° 3 5 x x C 3. Two ships leave a harbour, H, the first at 12:00 and the second at 12:30. The first ship sails on a course of N30°E at a speed of 20 km/h. The second ship sails on a course of S20°E at 15km/h. a) Determine the distance between the two ships at 14:30 to the nearest km. b) Determine the radar bearing from the first ship to the second ship. no diagram; messy diagram; small diagram; no labels in your diagram Common problems: Understand the problem. RHHS Mathematics Department Grade 11 Mathematics Page 2 of 3 Applications of Sine and Cosine Law (hints only) Date: F F 12 10 8 θ 6 4 2 H -2 50 km 30 ° 20 km /h for 2.5 h 30° x H 5 15 km /h for 2 h 130° 30 km -4 20° -6 -8 S S 4. Two concentric circles have centre C. Two points A and B are located on the smaller circle, with radius 10 cm, so that ∠ACB = 52° . Point M is located on the larger circle so that ∠CAM = 150° and ∠CBM = 135° . Determine the radius of the larger circle, accurate to one decimal. C ∠ ACB=52 ° ∠ CBM=135 ° C B B CP=10 P A ∠ CAM=150 ° P M CM=? Understand the problem. Then this diagram might be too small to be useful. Draw another “sterilized” diagram that helps you solve... RHHS Mathematics Department M CM = ??? A Grade 11 Mathematics Page 3 of 3 Applications of Sine and Cosine Law (hints only) Date: 5. Two fire towers are located 100 km apart on hills at points T and R. The bearing from T to R is northeast. A fire, F, is observed from tower T at N10°E and from tower R at N75°W. The town of Valleyview, at point V, is on a bearing of N25°E from T and S70°W from R. The observers report that the wind is blowing the fire directly toward Valleyview at 8 km/h. How much time do the town officials have in order to evacuate the town of Valleyview? Understand the problem. This one also needs 2 diagrams, each bigger than your hand! F N75°W N10°E R F S70°W 35° V R 25° V 135° N25°E TR=100 km 15° TR=100 km 20° 45° T Fig. 1: the diagram using the info given 45° T Fig. 2: Solve for the angles, as shown. 6. In right triangle ∆RST , ∠T = 90° . A point Q is located inside the triangle above base ST, such that SQ = 20 = TQ and ∠QST = 30° . If the measure of ∠R = 20° , determine the length of RQ, accurate to one decimal place. Understand the problem. Draw and label a diagram. If your labels are smaller than 10 pt font, draw it larger! Notice that ∆QTS is divided into two 30-60-90˚ triangles; producing TS. RHHS Mathematics Department
© Copyright 2025