Assignment 19
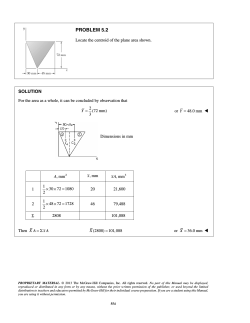
PROBLEM 5.100 For the machine element shown, locate the y coordinate of the center of gravity. SOLUTION For half-cylindrical hole, r = 1.25 in. 4(1.25) 3π = 1.470 in. yIII = 2 − For half-cylindrical plate, r = 2 in. 4(2) zIV = 7 + = 7.85 in. 3π V, in 3 y , in. z , in. y V , in 4 z V , in 4 3.5 –7.875 73.50 I Rectangular plate (7)(4)(0.75) = 21.0 –0.375 II Rectangular plate (4)(2)(1) = 8.0 1.0 2 8.000 16.00 III –(Half cylinder) (1.25) 2 (1) = 2.454 1.470 2 –3.607 –4.908 IV Half cylinder (2) 2 (0.75) = 4.712 –0.375 –1.767 36.99 V –(Cylinder) −π (1.25) 2 (0.75) = −3.682 1.381 –25.77 Σ 27.58 –3.868 95.81 − π 2 π 2 –0.375 –7.85 7 Y ΣV = Σ yV Y (27.58 in 3 ) = −3.868 in 4 Y = −0.1403 in. PROPRIETARY MATERIAL. © 2013 The McGraw-Hill Companies, Inc. All rights reserved. No part of this Manual may be displayed, reproduced or distributed in any form or by any means, without the prior written permission of the publisher, or used beyond the limited distribution to teachers and educators permitted by McGraw-Hill for their individual course preparation. If you are a student using this Manual, you are using it without permission. 672 PROBLEM 5.101 For the machine element shown, locate the y coordinate of the center of gravity. SOLUTION First assume that the machine element is homogeneous so that its center of gravity will coincide with the centroid of the corresponding volume. V , mm3 x , mm y , mm x V, mm 4 y V, mm 4 I (100)(18)(90) = 162, 000 50 9 8,100,000 1,458,000 II (16)(60)(50) = 48, 000 92 48 4,416,000 2,304,000 III π (12)2 (10) = 4523.9 105 54 475,010 244,290 IV −π (13) 2 (18) = −9556.7 28 9 –267,590 –86,010 Σ 204,967.2 12,723,420 3,920,280 We have Y ΣV = Σ yV Y (204,967.2 mm3 ) = 3,920, 280 mm 4 or Y = 19.13 mm PROPRIETARY MATERIAL. © 2013 The McGraw-Hill Companies, Inc. All rights reserved. No part of this Manual may be displayed, reproduced or distributed in any form or by any means, without the prior written permission of the publisher, or used beyond the limited distribution to teachers and educators permitted by McGraw-Hill for their individual course preparation. If you are a student using this Manual, you are using it without permission. 673 PROBLEM 5.118 Three brass plates are brazed to a steel pipe to form the flagpole base shown. Knowing that the pipe has a wall thickness of 8 mm and that each plate is 6 mm thick, determine the location of the center of gravity of the base. (Densities: brass = 8470 kg/m3; steel = 7860 kg/m3.) SOLUTION Since brass plates are equally spaced, we note that the center of gravity lies on the y-axis. x =z =0 Thus, π [(0.064 m) 2 − (0.048 m) 2 ](0.192 m) 4 = 270.22 × 10−6 m3 V= Steel pipe: m = ρ V = (7860 kg/m3 )(270.22 × 10−6 m3 ) = 2.1239 kg Each brass plate: 1 (0.096 m)(0.192 m)(0.006 m) = 55.296 × 10−6 m3 2 m = ρ V = (8470 kg/m3 )(55.296 × 10−6 m3 ) = 0.46836 kg V= Flagpole base: Σm = 2.1239 kg + 3(0.46836 kg) = 3.5290 kg Σ y m = (0.096 m)(2.1239 kg) + 3[(0.064 m)(0.46836 kg)] = 0.29382 kg ⋅ m Y Σm = Σ y m : Y (3.5290 kg) = 0.29382 kg ⋅ m Y = 0.083259 m Y = 83.3 mm above the base PROPRIETARY MATERIAL. © 2013 The McGraw-Hill Companies, Inc. All rights reserved. No part of this Manual may be displayed, reproduced or distributed in any form or by any means, without the prior written permission of the publisher, or used beyond the limited distribution to teachers and educators permitted by McGraw-Hill for their individual course preparation. If you are a student using this Manual, you are using it without permission. 694
© Copyright 2025