SAMPLE PROBLEMS
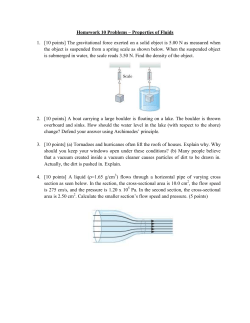
CALCULUS AB Section 3.7 (day 1): Optimization Problems, pg. 218 SAMPLE PROBLEMS 1. A garden is to be laid out in a rectangular area and protected by a chicken wire fence. What is the largest possible area of the garden if only 100 running feet of chicken wire is available for the fence? A Procedure for Solving Applied Maximum and Minimum Optimization Problems 1. 2. Draw an appropriate figure and label the quantities relevant to the problem. Find a formula for the quantity to be maximized or minimized. 3. Using the conditions stated in the problem to eliminated variables, express the quantity to be maximized or minimized as a function of one variable. 4. Find the interval of possible values for this variable from the physical restrictions in the problem. 5. If applicable, use the techniques of the preceding section (4.3) to obtain the maximum or minimum. 2. An open box is to be made from a 16-inch by 30-inch piece of cardboard by cutting out squares of equal size from the four corners and bending up the sides. What size should the squares be to obtain a box with the largest volume? 3. The following figure shows an offshore oil well located at a point W that is 5km from the closest point A on a straight shoreline. Oil is to be piped from W to a shore point B that is 8 km from A by piping it on a straight line under water from W to some shore point P between A and B via pipe along the shoreline. If the cost of laying pipe is $1,000,000/km under water and $500,000/km over land, where should the point P be located to minimize the cost of laying the pipe? W 5 km A P x B 8–x 8 km 4. Find a point in the curve y = x2 that is the closest to the point (18, 0). Application to economics Three functions of importance to an economist or manufacturer are: • C(x) = total cost of producing x units of a product during some period of time • R(x) = total revenue from selling x units of the product during the time period • P(x) = total profit obtained by selling x units of the product during the time period These are called, repetitively, the cost function, the revenue function, and the profit function. If all units produced are sold, then these are related by P(x) = R(x) - C(x) The total cost C(x) of producing x units can be expressed as a sum C(x) = a + M(x) Where “a” is a constant, called overhead, and M(x) is a function representing manufacturing cost. 5. A liquid form of penicillin manufactured by a pharmaceutical company is sold in bulk at a price of $200 ( R(x) = 200x )per unit. If the total production cost (in dollars) for x units is C ( x)=500,000+80 x + 0.003x 2 and if the production capacity of the firm is at most 30,000 units in a specified time, how many units of penicillin must be manufactured and sold in that time to maximize the profit?
© Copyright 2025